CBSE Class 9 Maths Triangles Notes:-
Introduction:
The concepts covered in Triangles Class 9 are important since they are carried on to higher education levels. For this reason, students are advised to learn the concept completely. The purpose of incorporating “Triangles” chapter in the NCERT textbook for Class 9 Maths is to teach students the following concepts:
- Triangle congruence
- SAS, ASA, SSS and RHS Congruence Rule
- Triangle Properties
- Triangle Inequalities
Congruent Triangles
In a pair of triangles if all three corresponding sides and three corresponding angles are exactly equal, then the triangles are said to be congruent.
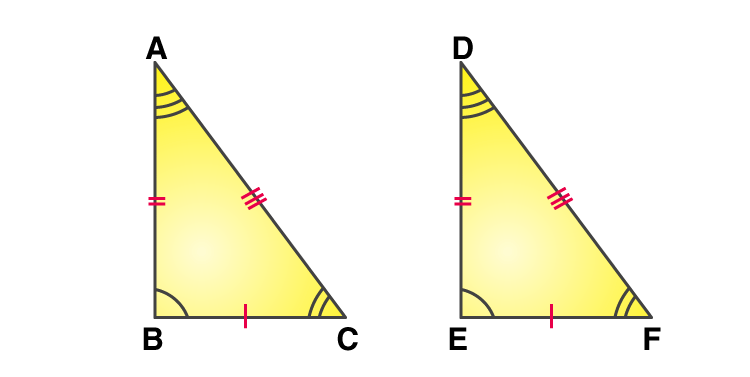
In congruent triangles, the corresponding parts are equal and are written as CPCT (Corresponding part of the congruent triangle).
Criteria for Congruency
Learning the congruence of triangles is very important to draw conclusions from the different polygon congruences. It has been used in real life, such as the construction of identical buildings, and so on. The following are the criteria for the congruency of the triangles.
- SAS
- ASA
- AAS
- SSS
- RHS
symbolically, it is expressed as ΔABC ≅ ΔXYZ
SSS Criteria for Congruency
- If the three sides of one triangle are equal to the three sides of another triangle, then the two triangles are congruent.
- If all sides are exactly the same, then their corresponding angles must also be exactly the same.
SAS Criteria for Congruency
– Axiom: Two triangles are congruent if two sides and the included angle of one triangle are equal to the corresponding sides and the included angle of the other triangle.
ASA Criteria for Congruency
– Two triangles are congruent if two angles and the included side of one triangle are equal to the corresponding two angles and the included side of the other triangle
AAS Criteria for Congruency
– Two triangles are said to be congruent to each other if two angles and one side of one triangle are equal to two angles and one side of the other triangle.
Why Are SSA and AAA Congruency rules Not Valid?
- SSA test is not a valid test for congruency as the angle is not included between the pairs of equal sides.
- The AAA test also is not a valid test as even though 2 triangles can have all three same angles, the sides can be of differing lengths. This becomes a test for similarity (AA).

Angles of a triangle
RHS Criteria for Congruency
- If in two right triangles, the hypotenuse and one side of one triangle are equal to the hypotenuse and one side of the other triangle, then the two triangles are congruent.
- RHS stands for Right angle – Hypotenuse – Side.
Properties of Isosceles Triangle
Isosceles Triangle Property
Angles opposite to equal sides of an isosceles triangle are equal.
Converse of Isosceles Triangle Property
The sides opposite the equal angles of a triangle are equal.
Inequalities in Triangles
Relationship between unequal sides of the triangle and the angles opposite to it.
If 2 sides of a triangle are unequal, then the angle opposite to the longer side will be larger than the angle opposite to the shorter side.
Triangle Inequality
The sum of the lengths of any two sides of a triangle must be greater than the third side.